We investigate the evolution of Bessel–Gaussian (BG) optical beams in using the fractional Schr?dinger equation (FSE) without potential or with nonlocal nonlinear media, theoretically and numerically. We find that, as the propagation distance increases, the linear propagation dynamics of the 1D BG beams undergo an initial compression phase, after which each of the beams splits into two sub-beams; these sub-beams then separate from each other, forming a saddle shape as the propagation distance continues to increase; in addition, their interval also increases linearly with propagation distance. However, when the nonlocal nonlinear term is included in the FSE, 1D BG beams follow a zigzag trajectory in real space, which corresponds to a modulated anharmonic oscillation in momentum space. In the 2D case, the input chirped BG beam first evolves into a filament in real space and then into a ring structure; if the input is a superposed BG beam carrying orbital angular momentum, the rule fulfilled in evolution is similar to that for a single one, and it forms a funnel-like structure, with periodic inversion and variable rotation.
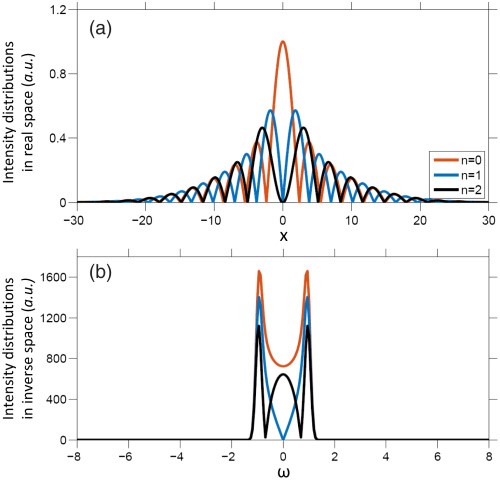
Intensity distributions of the BG beam for different orders $n$ (a) in real space and (b) in momentum space. The other parameters are $a = 1$ and $\sigma = 1$.