Competing nonlinearities, such as the cubic (Kerr) and quintic nonlinear terms whose strengths are of opposite signs (the coefficients in front of the nonlinearities), exist in various physical media (in particular, in optical and matter-wave media). A benign competition between self-focusing cubic and self-defocusing quintic nonlinear nonlinearities (known as cubic–quintic model) plays an important role in creating and stabilizing the self-trapping of D-dimensional localized structures, in the contexts of standard nonlinear Schr?dinger equation. Jianhua Zeng’s research team incorporates an external periodic potential (linear lattice) into this model and extend it to the space-fractional scenario that begins to surface in very recent years—the nonlinear fractional Schr?dinger equation (NLFSE), therefore obtaining the cubic–quintic or the purely quintic NLFSE, and investigate the propagation and stability properties of self-trapped modes therein. Two types of one-dimensional localized gap modes are found, including the fundamental and dipole-mode gap solitons. Employing the techniques based on the linear-stability analysis and direct numerical simulations, they get the stability regions of all the localized modes; and particularly, the anti-Vakhitov–Kolokolov criterion applies for the stable portions of soliton families generated in the frameworks of quintic-only nonlinearity and competing cubic–quintic nonlinear terms.
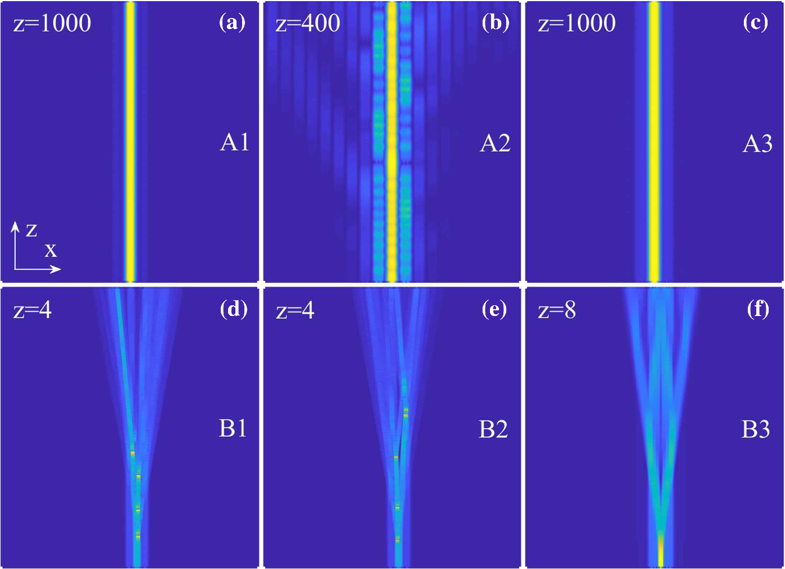
(Original research article "Nonlinear Dynamics, Volume 98, Issue 2, pp 985–995. https://doi.org/10.1007/s11071-019-05240-x)