The existence and stability of stable bright solitons in one-dimensional (1D) fractional media with a spatially periodical modulated Kerr nonlinearity (nonlinear lattice), supported by the recently introduced nonlinear fractional Schr?dinger equation, are demonstrated by means of the linear-stability analysis and in direct numerical simulations. Both 1D fundamental and multipole solitons (in forms of dipole and tripole ones) are found, which occupy one or three cells of the nonlinear lattice, respectively, depending on the soliton’s power. Jianhua Zeng’s research team recently found that the profiles of the predicted soliton families are impacted intensely by the Lévy index ??, and so are their stability. The soliton families are stable if ?? exceeds a threshold value, below which the balance between fractional-order diffraction and the spatially modulated focusing nonlinearity will be broken.

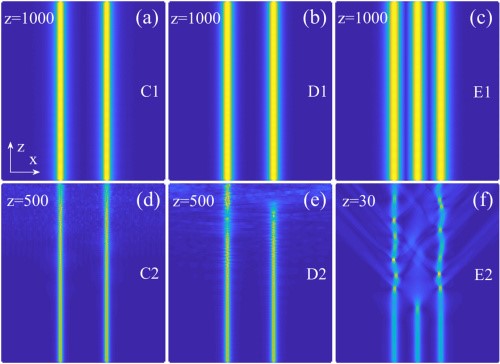
（Original research article "Optics Letters Vol. 44, Issue 11, pp. 2661-2664 (2019) https://doi.org/10.1364/OL.44.002661）